NormDis, two-way calculator model for the normal probability distribution with graphics
  Entirely free download and use of software


NormDis is a calculator program using a model application (app) for the normal probability distribution.

The values of a random variable X may follow a normal probability distribution (NPD).

The applicabe NPD is found from the mean (average) M of the X-values and their standard deviation (SD).

With the NPD one can calculate the cumulative probability (CP) of any reference X-value (Xr) or, reversely, one can find the Xr of any CP.

The CP(Xr) is the probability (P) that an X-value is less than the reference value Xr: CP(Xr) = P(X < Xr).

The probability (P) that X occurs between a lower limit (L) and an upper limit (U) can be found from: P(L < X < U) = CP(U) - CP(L).

The normal distribution is used in the Z - test for significance testing of observations.


            Screenprint: two-way calculator application for the normal probability distribution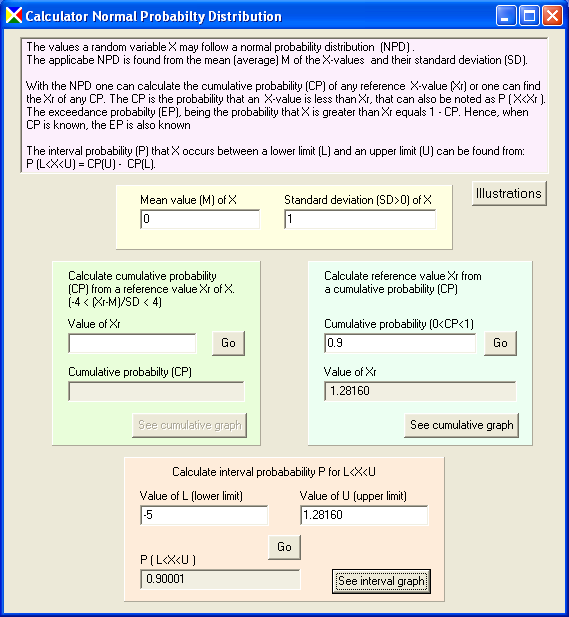

                    screenprint normdis    

Experiences: For improvement, I am interested to learn about your experiences with NormDis. For this there is a contact form.


Download:

"NormDis"
normal distribution
calculator




Go to:


software
& models


articles
& manuals


reports
case studies


FAQ's
& papers


home
page
 

MATHEMATICS
There is no analytical solution to the cumulative normal distribution. The NormDis model program uses Hastings' numerical approximation as described in this Wikipedia article in the section "Numerical approximations for the normal CDF" referring to Zelen & Severo (1964).

PROBABILITY OF EXCEEDANCE
The exceedance probability (EP), being the probability that X is greater than Xr equals 1 - CP. Hence, when CP is known, the EP is also known.


here comes an image

NormDis software graphics in accordance to data in the above screenprint of the z-test calculator for the normal probability distribution

            cumulative normal distribution
The NormDis calculator program uses the cumulative normal probability distribution for the z - test 		            normal density distribution
The NormDis calculator software uses the normal probability density distribution for the z - test